 |
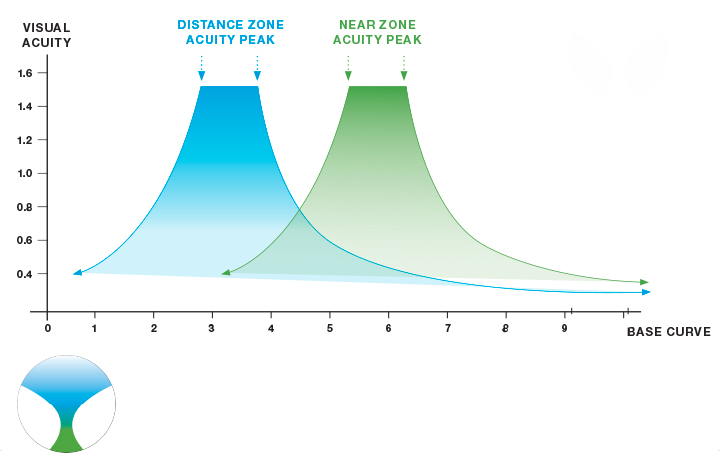
Digital lens technology has allowed labs to create progressive lenses out of single vision lens blanks. But pairing lens power to ideal base curve in this situation is complicated — and compromises are inevitable. A progressive lens has many powers, hence it has many ideal base curves, increasing in diopter from top to bottom. The distance zone power calls for a flatter base curve, while the near zone power calls for a steeper
base curve. |
However, when a free-form progressive lens is processed from a single-vision lens blank, the various powers must share a single base curve, one that may not be ideal for the near zone. The Camber lens offers an elegant solution that represents the next step in digital progressive technology. Camber lens' front surface innovation offers wearers spacious, more comfortable fields of vision, in both the distance zone and reading area. |
Multiple Ideal Base Curves
A progressive lens has many powers from distance to near, hence it has many ideal base curves, increasing in diopter from top to bottom.